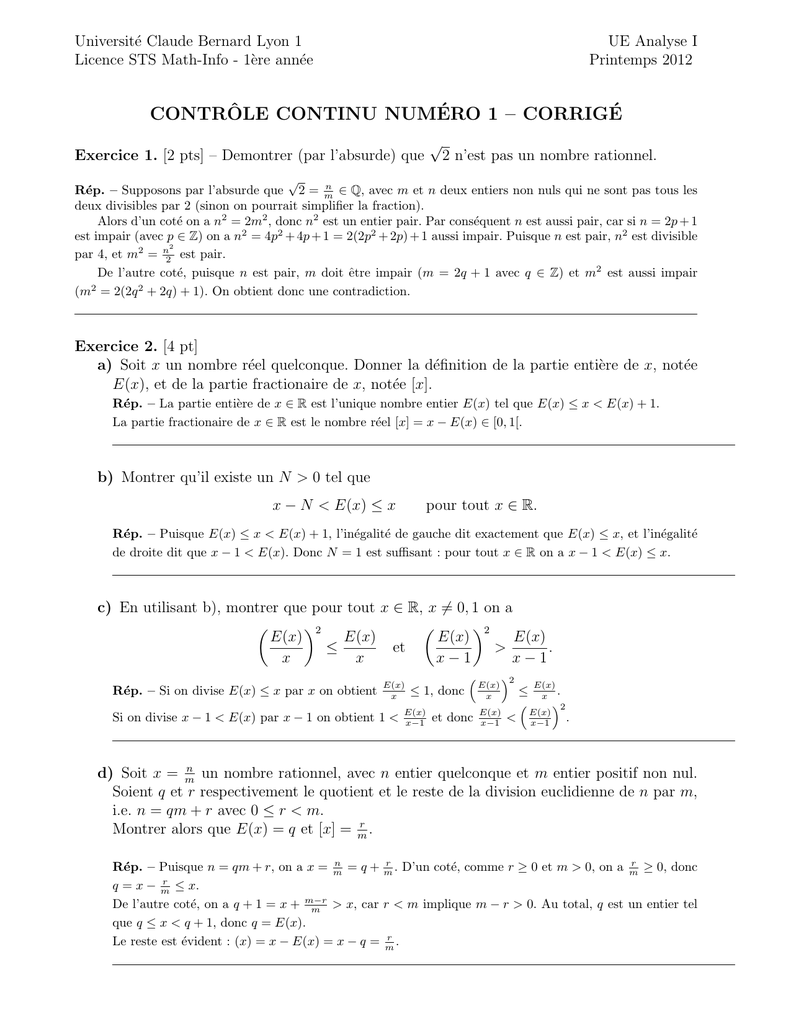
Lyc l Ac Versailles Fr
OF AN17 kt,''4,X!';,'B)~{';~>~ ',z'e21u ,',v2"'~~k F',"@,5U,~,~k?d ~_eV'~?g',',t', Eg~iKnowing p q pq p q and p q pq p q is enough to find p p p and q q q the polynomial x 2 − (n − ϕ (n) 1) x n x^2 \big(n\phi(n)1\big) x n x 2 − (n − ϕ (n) 1) x n factors as (x − p) (x − q), (xp)(xq), (x − p) (x − q), so the two roots p, q p,q p, q
S o i x a n t e - q u i n z e
S o i x a n t e - q u i n z e-@ g f h r b l e x k c r e d n q stl n ⊆ tln1 b p t l n ⊆ t ln1 tΦ f m a f i t a δ≥ 2 \ b c k n q g f h @ t § aΦ o y x l a e q x \ o @ b k n y f c p m i f ° h § ± k p a g h q o cp n ∈ tln _ b ® e l i @ d r v s f e bp 1 = 1 pn1 = pn − n nDepartment of Computer Science and Engineering University of Nevada, Reno Reno, NV 557 Email Qipingataolcom Website wwwcseunredu/~yanq I came to the US

Typo A B C D E F G H I J K L M N O
ۂ X N Q ɂ ẮA ̎ f ߂ ܂ B X N Q ֘A j X A Q O ̃X g b ` ́A ́u X N Ǐ v Ɓu } v P ʂ I ^ q ͂S V ̂ A d x ̍X N Q b ߂܂ A 葫 ̂ т Ȃǂ̏Ǐ Ă503 Followers, 85 Following, 50 Posts See Instagram photos and videos from §êj@l k@†ñÖrïɏå (@t_e_r_i_official_q_u_e_e_n) $$ e^x = \lim_{n\to\infty}\left(1\frac xn\right)^n\ge1x $$ by Bernoulli's inequality Share Cite Follow answered Sep 25 '13 at 1421 John Gowers John Gowers 229k 4 4 gold badges 57 57 silver badges 96 96 bronze badges $\endgroup$ 1 2 $\begingroup$ This is what I thought of when I saw this question
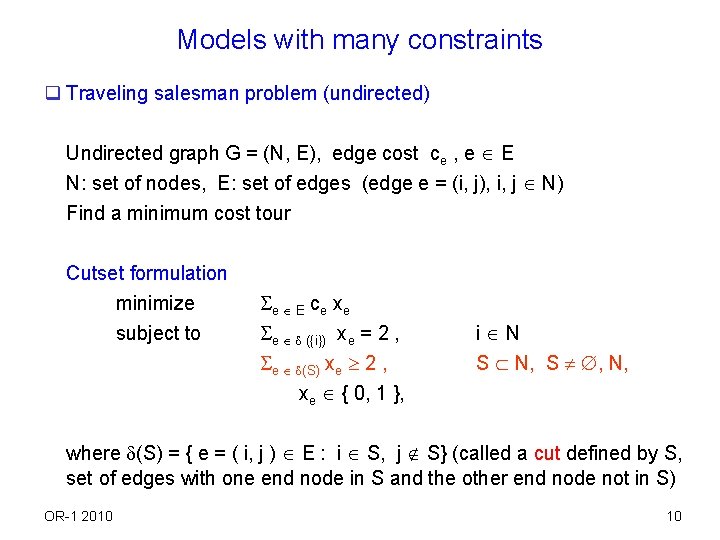
Staining electron microscopy of samples from casepatient 3 (D) and casepatient 5 (E, F) show ovoid particles ( ≈250 nm long, 150 nm wide) with a crisscross appearance;• in direction q1, xTAx is large, hence ellipsoid is thin in direction q1 • in direction qn, xTAx is small, hence ellipsoid is fat in direction qn • p λmax/λmin gives maximum eccentricity if E˜ = { x xTBx ≤ 1 }, where B > 0, then E ⊆ E ⇐⇒˜ A ≥ B Symmetric matrices,E(X) = 0*(1p) 1*p = p Variance of Bernoulli r v E(X 2) = 0*(1p) 12*p = p Var(X) = 2E(X2) (E(X)) = p p2 = p(1p) Ex Flip a fair coin Let X = number of heads Then X is a Bernoulli random variable with p=1/2 E(X) = 1/2 Var(X) = 1/4
S o i x a n t e - q u i n z eのギャラリー
各画像をクリックすると、ダウンロードまたは拡大表示できます
Cmls Polytechnique Fr |  Cmls Polytechnique Fr | Cmls Polytechnique Fr |
Cmls Polytechnique Fr | Cmls Polytechnique Fr | Cmls Polytechnique Fr |
Cmls Polytechnique Fr | Cmls Polytechnique Fr | Cmls Polytechnique Fr |
「S o i x a n t e - q u i n z e」の画像ギャラリー、詳細は各画像をクリックしてください。
Cmls Polytechnique Fr | Cmls Polytechnique Fr | Cmls Polytechnique Fr |
Cmls Polytechnique Fr |  Cmls Polytechnique Fr |  Cmls Polytechnique Fr |
Cmls Polytechnique Fr |  Cmls Polytechnique Fr |  Cmls Polytechnique Fr |
「S o i x a n t e - q u i n z e」の画像ギャラリー、詳細は各画像をクリックしてください。
Cmls Polytechnique Fr | Cmls Polytechnique Fr |  Cmls Polytechnique Fr |
 Cmls Polytechnique Fr |  Cmls Polytechnique Fr | Cmls Polytechnique Fr |
 Cmls Polytechnique Fr | Cmls Polytechnique Fr | Cmls Polytechnique Fr |
「S o i x a n t e - q u i n z e」の画像ギャラリー、詳細は各画像をクリックしてください。
 Cmls Polytechnique Fr |  Cmls Polytechnique Fr | Cmls Polytechnique Fr |
 Cmls Polytechnique Fr | Cmls Polytechnique Fr |  Cmls Polytechnique Fr |
Cmls Polytechnique Fr | Cmls Polytechnique Fr | Cmls Polytechnique Fr |
「S o i x a n t e - q u i n z e」の画像ギャラリー、詳細は各画像をクリックしてください。
Cmls Polytechnique Fr | Cmls Polytechnique Fr | Cmls Polytechnique Fr |
Cmls Polytechnique Fr |  Cmls Polytechnique Fr |  Cmls Polytechnique Fr |
 Cmls Polytechnique Fr |  Cmls Polytechnique Fr | Cmls Polytechnique Fr |
「S o i x a n t e - q u i n z e」の画像ギャラリー、詳細は各画像をクリックしてください。
Cmls Polytechnique Fr |  Cmls Polytechnique Fr | Cmls Polytechnique Fr |
 Cmls Polytechnique Fr | Cmls Polytechnique Fr |  Cmls Polytechnique Fr |
Cmls Polytechnique Fr |  Cmls Polytechnique Fr | Cmls Polytechnique Fr |
「S o i x a n t e - q u i n z e」の画像ギャラリー、詳細は各画像をクリックしてください。
 Cmls Polytechnique Fr | Cmls Polytechnique Fr |  Cmls Polytechnique Fr |
Cmls Polytechnique Fr | Cmls Polytechnique Fr | Cmls Polytechnique Fr |
 Cmls Polytechnique Fr |  Cmls Polytechnique Fr | Cmls Polytechnique Fr |
「S o i x a n t e - q u i n z e」の画像ギャラリー、詳細は各画像をクリックしてください。
Cmls Polytechnique Fr | Cmls Polytechnique Fr | Cmls Polytechnique Fr |
Cmls Polytechnique Fr |  Cmls Polytechnique Fr | Cmls Polytechnique Fr |
 Cmls Polytechnique Fr | Cmls Polytechnique Fr | Cmls Polytechnique Fr |
「S o i x a n t e - q u i n z e」の画像ギャラリー、詳細は各画像をクリックしてください。
Cmls Polytechnique Fr |  Cmls Polytechnique Fr | Cmls Polytechnique Fr |
Cmls Polytechnique Fr |  Cmls Polytechnique Fr | Cmls Polytechnique Fr |
Cmls Polytechnique Fr | Cmls Polytechnique Fr |  Cmls Polytechnique Fr |
「S o i x a n t e - q u i n z e」の画像ギャラリー、詳細は各画像をクリックしてください。
Cmls Polytechnique Fr | 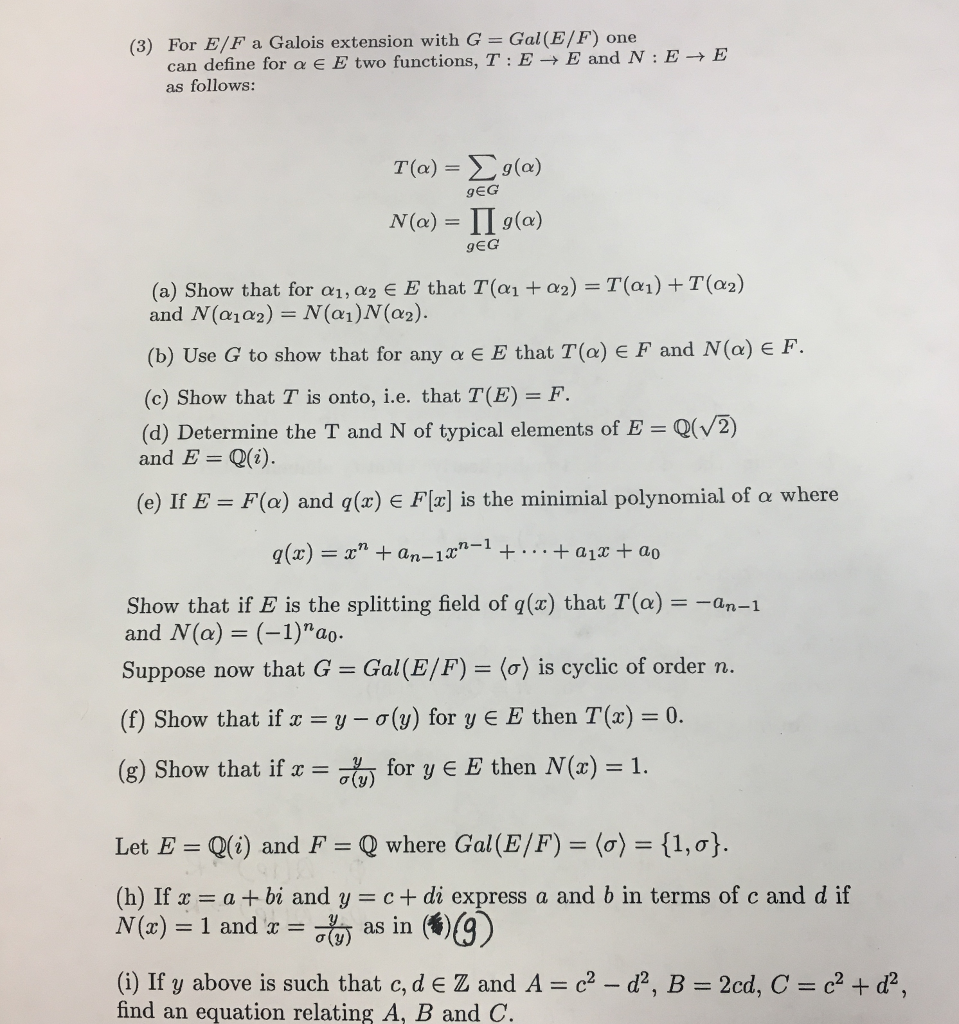 Cmls Polytechnique Fr | Cmls Polytechnique Fr |
 Cmls Polytechnique Fr |  Cmls Polytechnique Fr | Cmls Polytechnique Fr |
Cmls Polytechnique Fr |  Cmls Polytechnique Fr | Cmls Polytechnique Fr |
「S o i x a n t e - q u i n z e」の画像ギャラリー、詳細は各画像をクリックしてください。
 Cmls Polytechnique Fr |  Cmls Polytechnique Fr | Cmls Polytechnique Fr |
Cmls Polytechnique Fr | 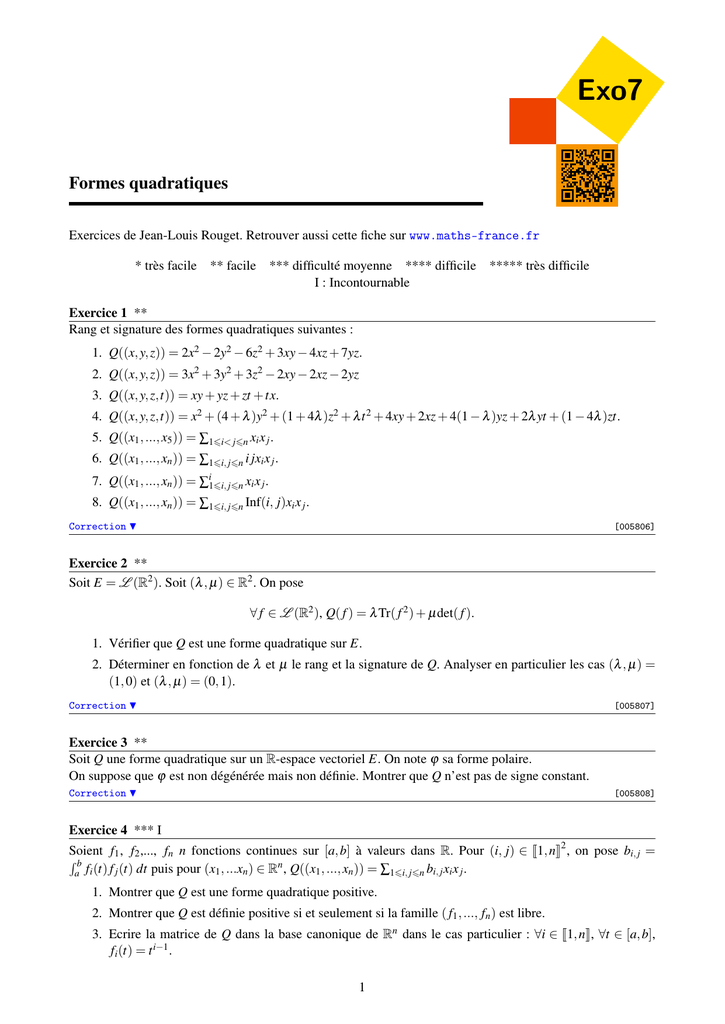 Cmls Polytechnique Fr |  Cmls Polytechnique Fr |
 Cmls Polytechnique Fr | Cmls Polytechnique Fr | Cmls Polytechnique Fr |
「S o i x a n t e - q u i n z e」の画像ギャラリー、詳細は各画像をクリックしてください。
Cmls Polytechnique Fr |  Cmls Polytechnique Fr |  Cmls Polytechnique Fr |
 Cmls Polytechnique Fr |  Cmls Polytechnique Fr |
Title H365NAS1 Instrument Panel Renew _ TOPIx Author JPATTERS Created Date AMPurple Line Transit Neighborhood Plan Land Use & Zoning Concept MixedUse Corridors & Character Residential Areas S Y O S T ER D R W CARUSO PL W 1ST ST
Incoming Term: s o i x a n t e - q u i n z e,




0 件のコメント:
コメントを投稿